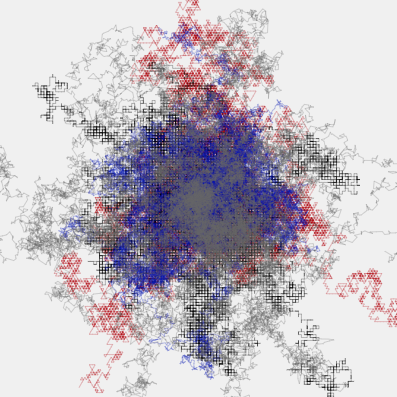
“Anomalous Itinerary”
Lévy flights
This explorable illustrates the properties of a class of random walks known as Lévy flights. To get the most out of this explorable, you may want to check out the explorable Albert & Carl Friedrich on ordinary random walks and diffusion first.
“Albert & Carl Friedrich”
Random Walks & Diffusion
This explorable illustrates the geometric and dynamic properties of the physical process of diffusion and its intimate relation to a mathematical object known as a random walk. It also illustrates graphically the implications of the central limit theorem that explains why we so often (normally) observe Gaussian distributions in nature. In the context of random walks this means that in the long run and from a great distance the paths of different types of walks become statistically indistiguishable.
“Flock'n Roll”
Collective behavior and swarming
This explorable illustrates of an intuitive dynamic model for collective motion (swarming) in animal groups. The model can be used to describe collective behavior observed in schooling fish or flocking birds, for example. The details of the model are described in a 2002 paper by Iain Couzin and colleagues.
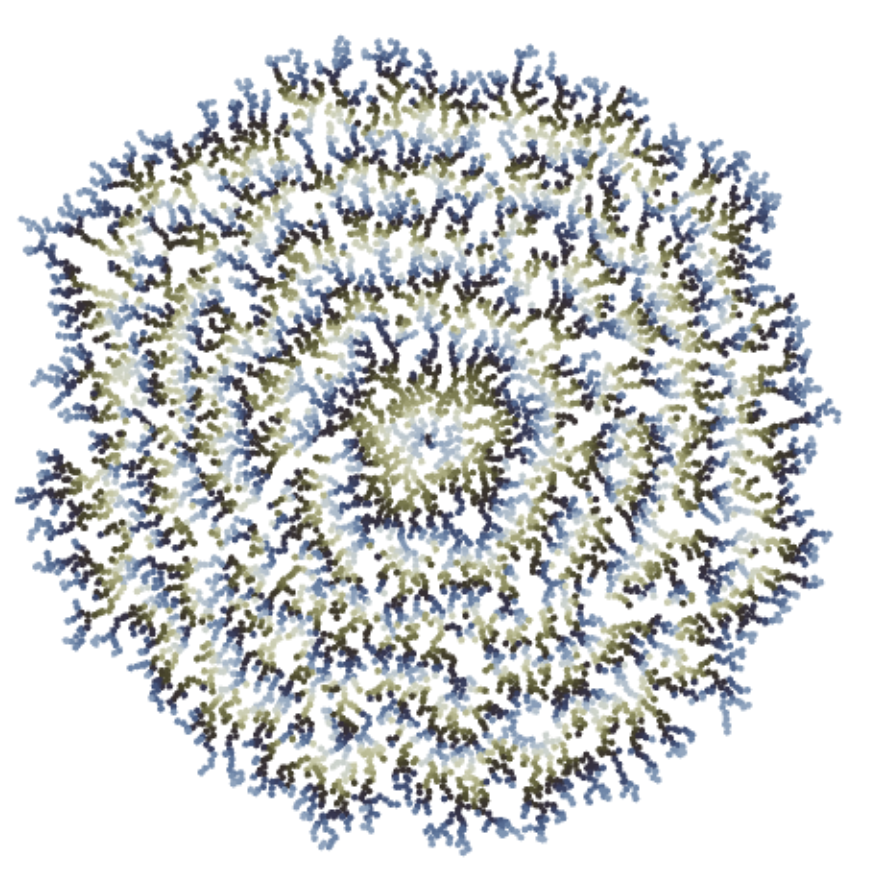
“Particularly Stuck”
Diffusion Limited Aggregation
This explorable illustrates a process known as diffusion-limited aggregation (DLA). It’s a kinetic process driven by randomly diffusing particles that gives rise to fractal structures, reminiscent of things we see in natural systems. The process has been investigated in a number of scientific studies, e.g. the seminal paper by Witten & Sander.