“Spin Wheels”
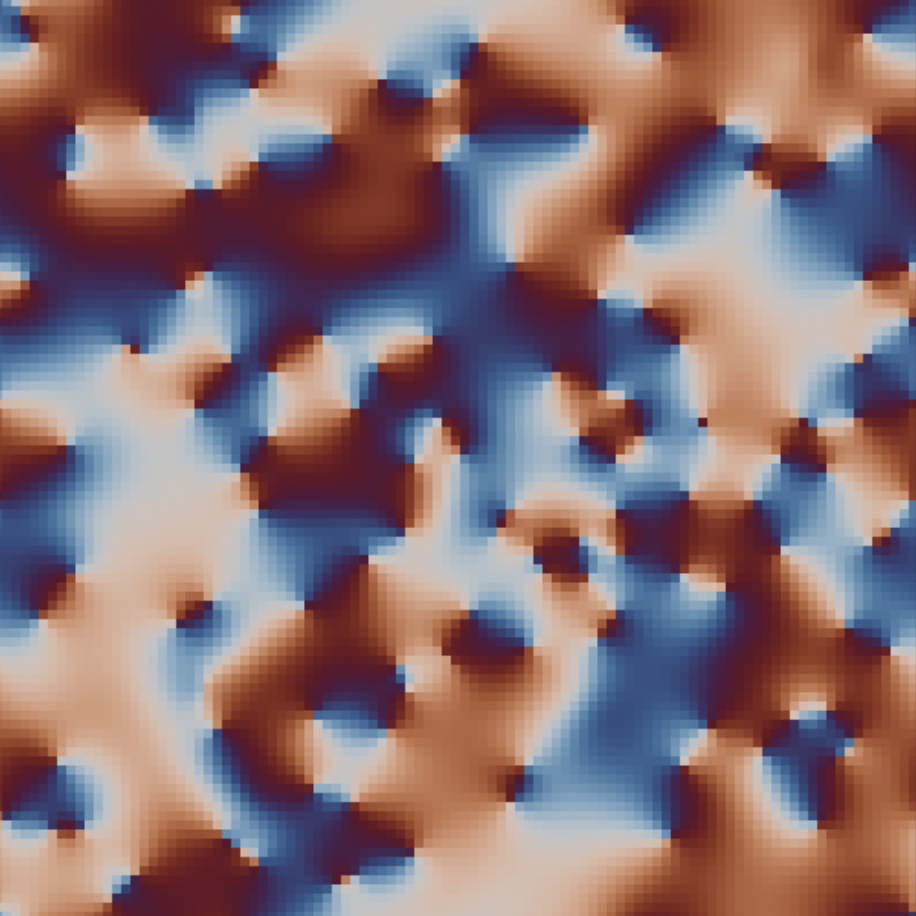
Phase-coupled oscillators on a lattice
This explorable illustrates pattern formation, interesting and beautiful properties of oscillators that are spatially arranged on a lattice and interact with their neighbors. Oscillators and their interaction are described by the famous Kuramoto Model for phase coupled oscillators. The model is amazing, because on one hand it is conceptually quite simple, on the other it holds a number of unexpected dynamical secrets that you can discover here.
“Swårmalätørs”
Oscillators that sync and swarm
This explorable illustrates how remarkable spatio-temporal patterns can emerge when two dynamical phenomena, synchronization and collective motion, are combined. In the model, a bunch of oscillators move around in space and interact. Each oscillator has an internal oscillatory phase. An oscillator’s movement and change of internal phase both depend on the positions and internal phases of all other oscillators. Because of this entanglement of spatial forces and phase coupling the oscillators are called swarmalators.
“Janus Bunch”
Dynamics of two-phase coupled oscillators
“Dr. Fibryll & Mr. Glyde”
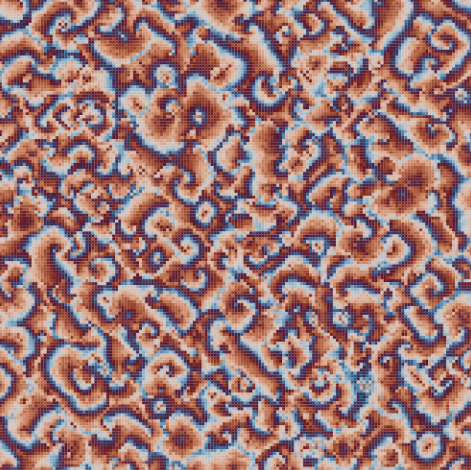
Pulse-coupled oscillators
This explorable illustrates pattern formation in excitable media. The example explored here in a system of pulse-coupled oscillators that are arranged on a two-dimensional lattice and interact with their neighbors by delivering excitatory pulses to them and receiving them in return. This model is sometimes used to study synchronization and can capture the dynamics of activation in layers of neurons as well as the spatial patterns of signaling molecules that play a role in microbial aggregation processes.
“Stranger Things”
Strange attractors
This explorable illustrates the structure and beauty of strange attractors of two-dimensional discrete maps. These maps generate sequences of pairs of number $(x_n,y_n)$ where the index $n=0,1,2,…$ denotes the step of the iteration process that starts at the point $(x_0,y_0)$. The map is defined by two functions $f(x,y)$ and $g(x,y)$ that determine the point $(x_{n+1},y_{n+1})$ given $(x_{n},y_{n})$:
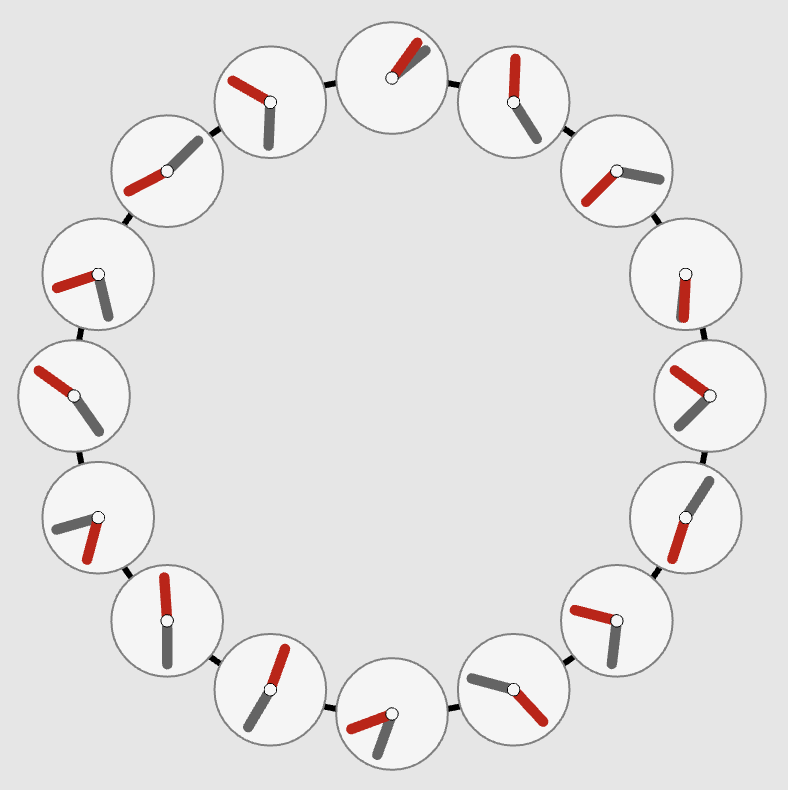
“Ride my Kuramotocycle!”
The Kuramoto model
This explorable illustrates the Kuramoto model for phase coupled oscillators. This model is used to describe synchronization phenomena in natural systems, e.g. the flash synchronization of fire flies or wall-mounted clocks.
“Lotka Martini”
The Lotka-Volterra model
This explorable illustrates the dynamics of a predator-prey model on a hexagonal lattice. In the model a prey species reproduces spontaneously but is also food to the predator species. The predator requires the prey for reproduction. The system is an example of an activator-inhibitor system, in which two dynamical entities interact in such a way that the activator (in this case the prey) activates the inhibitor (the predator) that in turn down-regulates the activator in a feedback loop. Activator-inhibitor systems often exhibit oscillatory behavior, like the famous Lotka-Volterra System, a paradigmatic model for predator prey dynamics.
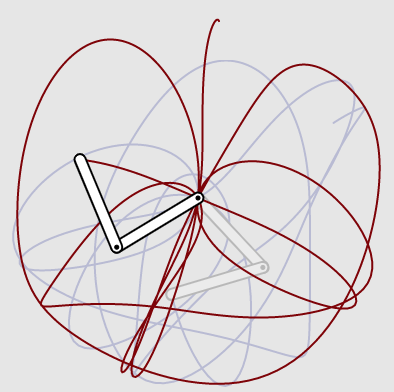
“Double Trouble”
The double pendulum
This explorable illustrates the beautiful dynamical features of the double pendulum, a famous idealized nonlinear mechanical system that exhibits deterministic chaos. The double pendulum is essentially two simple pendula joined by a bearing. It’s a classic complex system in which a simple setup generates rich and seemingly unpredictable behavior. The only force that is acting on it is gravity. There’s no friction.
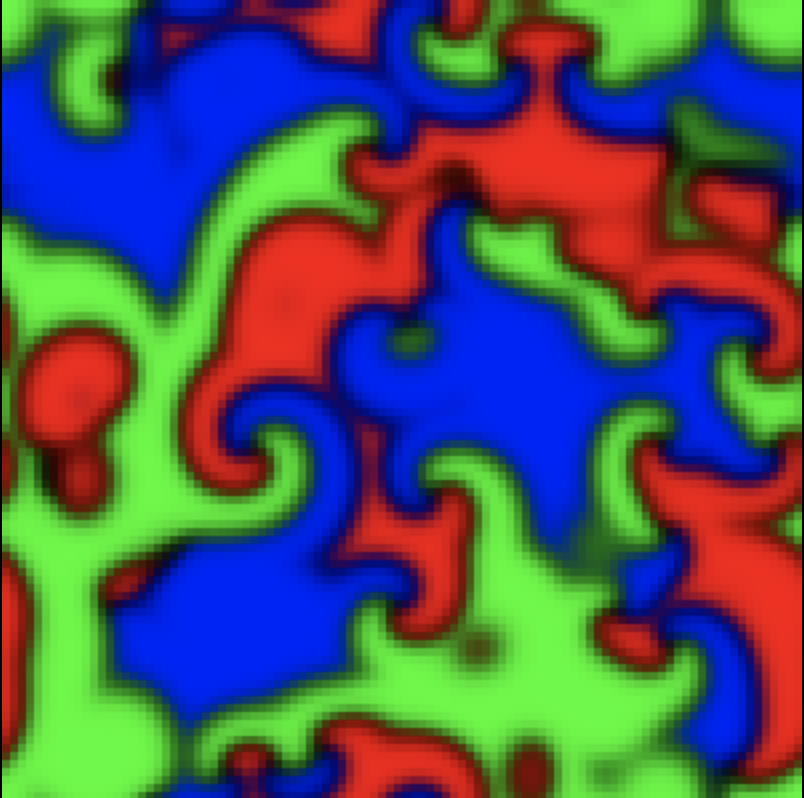
“Cycledelic”
The spatial rock-paper-scissors game
This explorable of a pattern forming system is derived from a model that was designed to understand co-existance of cyclicly interacting species in a spatially extended model ecosystem. Despite its simplicity, it can generate a rich set of complex spatio-temporal patterns depending on the choice of parameters and initial conditions.
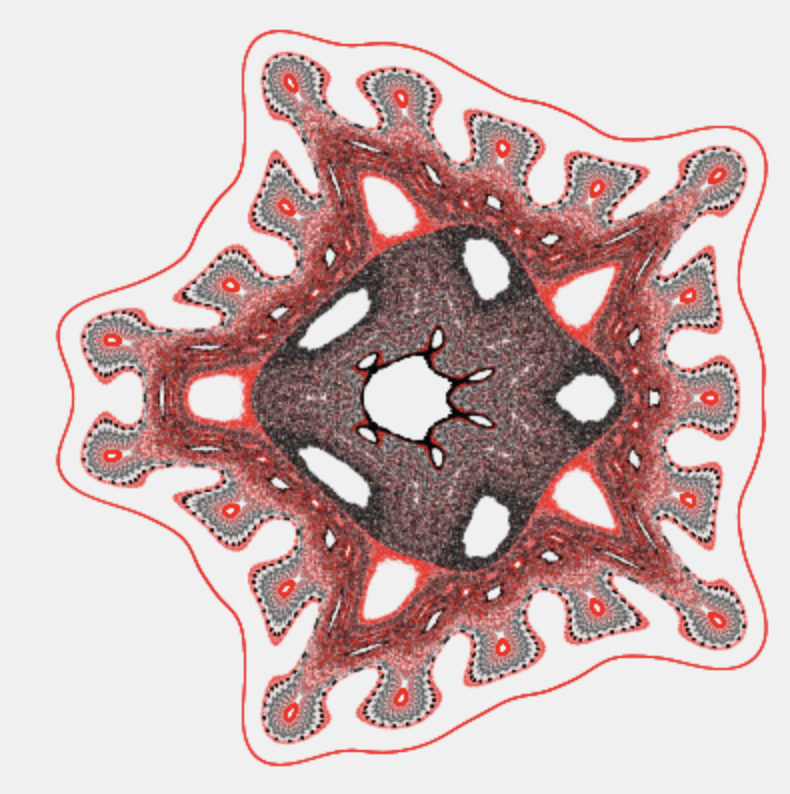
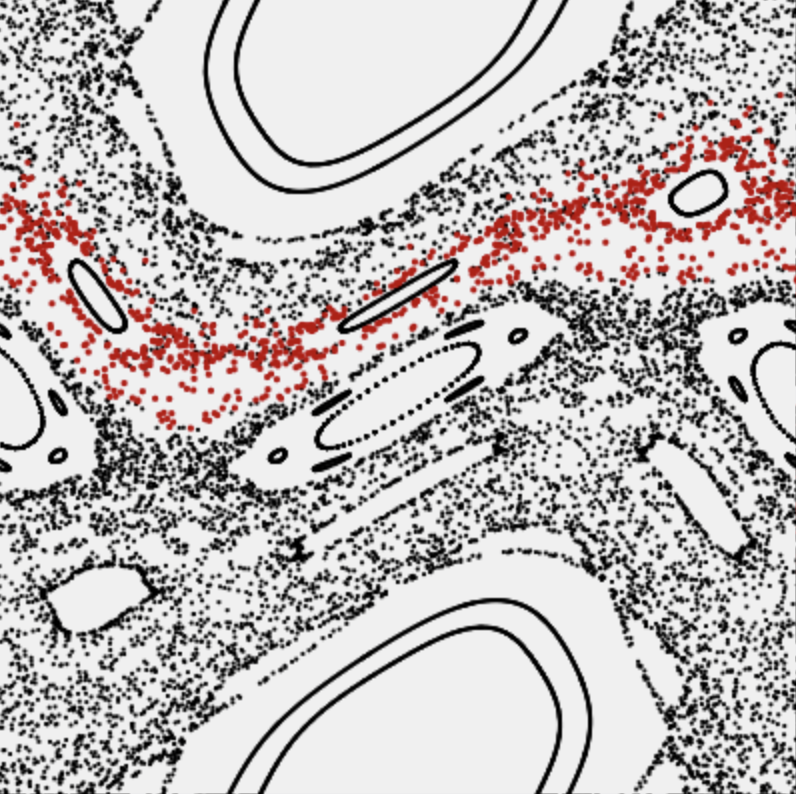
“Kick it like Chirikov”
The kicked rotator (standard map)
In this explorable you can investigate the dynamics of a famous two-dimensional, time discrete map, known as the standard or Chirikov–Taylor map, one of the most famous dynamical systems that exhibits deterministic chaos. It is almost identical to the “Kicked Rotator”, an idealized physical system in which a rotating rod is periodically kicked by an external force.