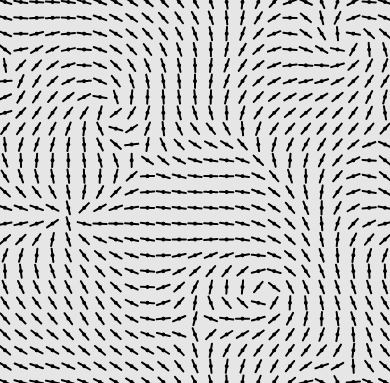
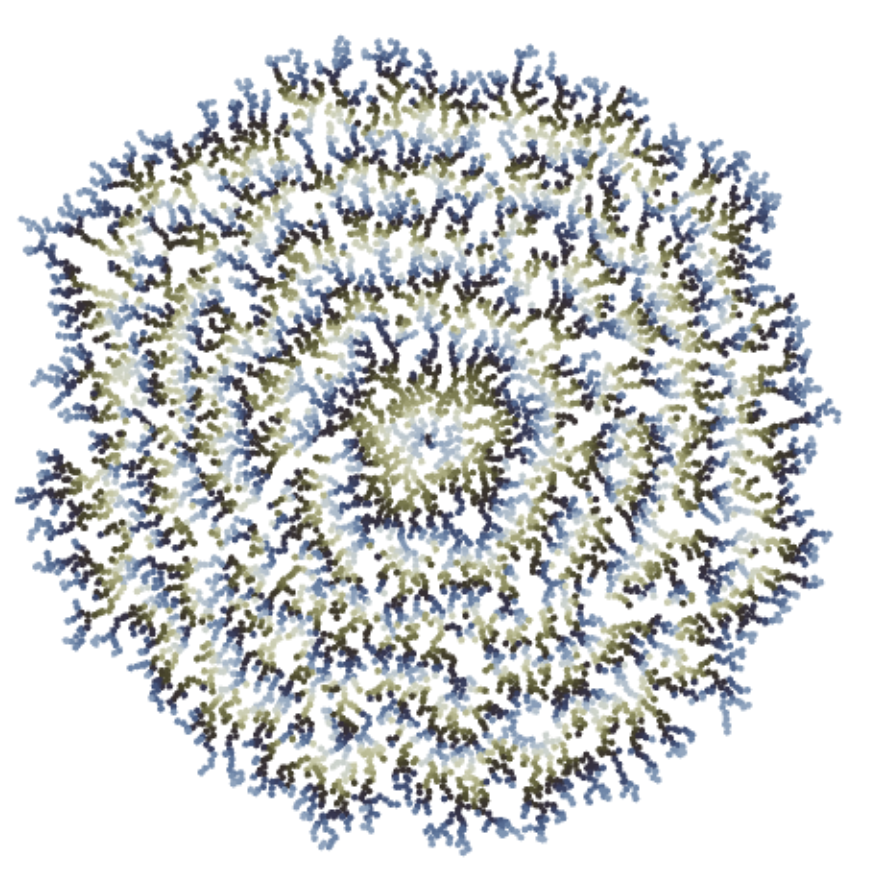
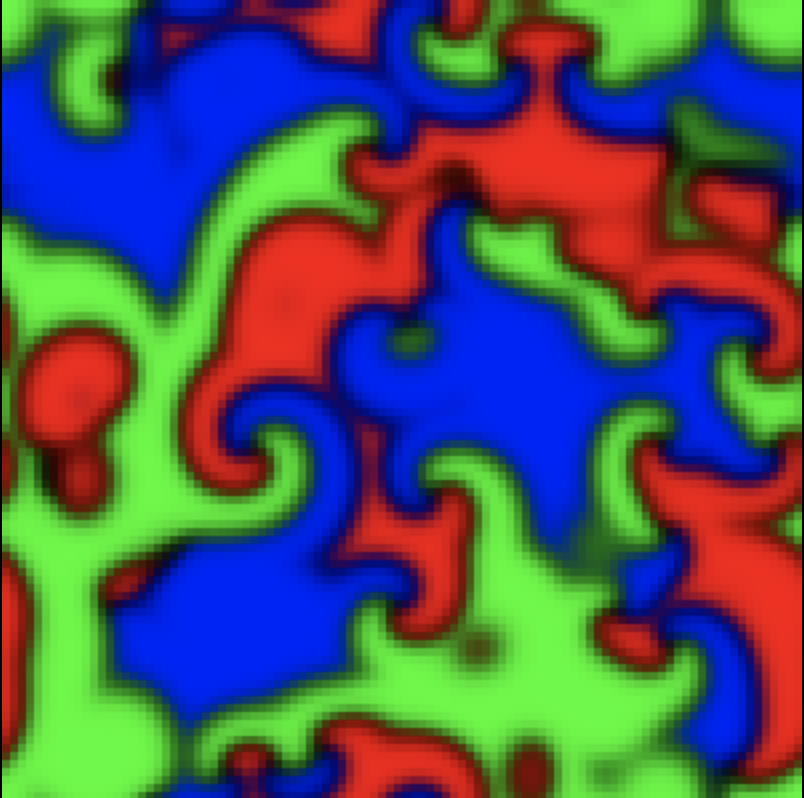
“Spin Wheels”
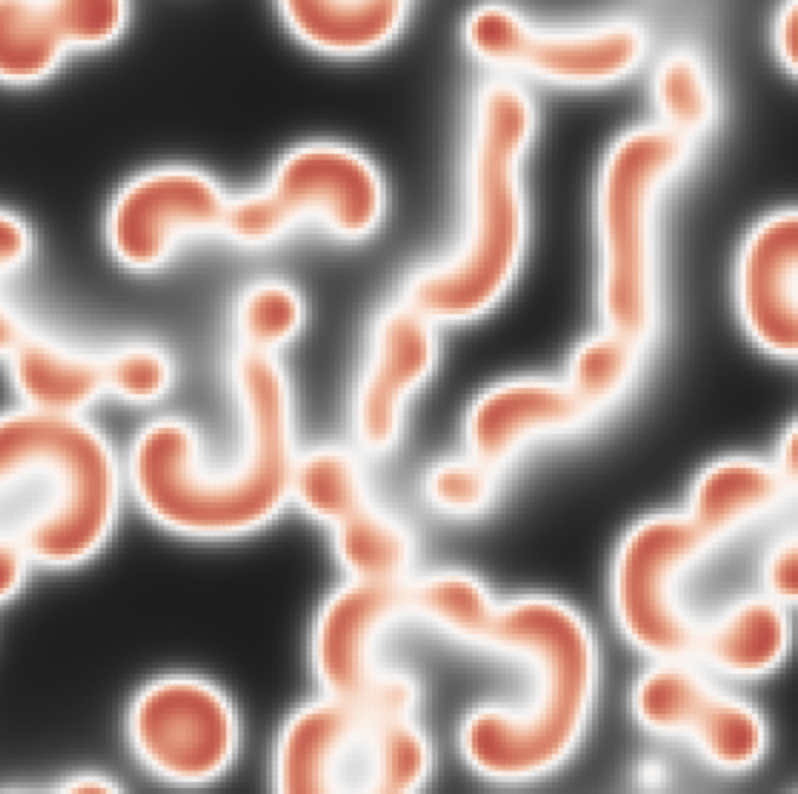
Phase-coupled oscillators on a lattice
This explorable illustrates pattern formation, interesting and beautiful properties of oscillators that are spatially arranged on a lattice and interact with their neighbors. Oscillators and their interaction are described by the famous Kuramoto Model for phase coupled oscillators. The model is amazing, because on one hand it is conceptually quite simple, on the other it holds a number of unexpected dynamical secrets that you can discover here.
“Eigenartig”
The spatial hypercycle model
This explorable illustrates the dynamics of the famous hypercycle model. It was originally conceived by Peter Schuster and Nobel laureate Manfred Eigen (1927-2019) in 1979 to investigate the chemical basis of the origin of life. Because living things make copies of themselves, in the beginning complex chemicals like polymer chains, including small RNA molecules (see e.g. RNA-world), had to acquire the ability to catalyse their own synthesis from smaller parts, e.g. single nucleotides.
“Come Together”
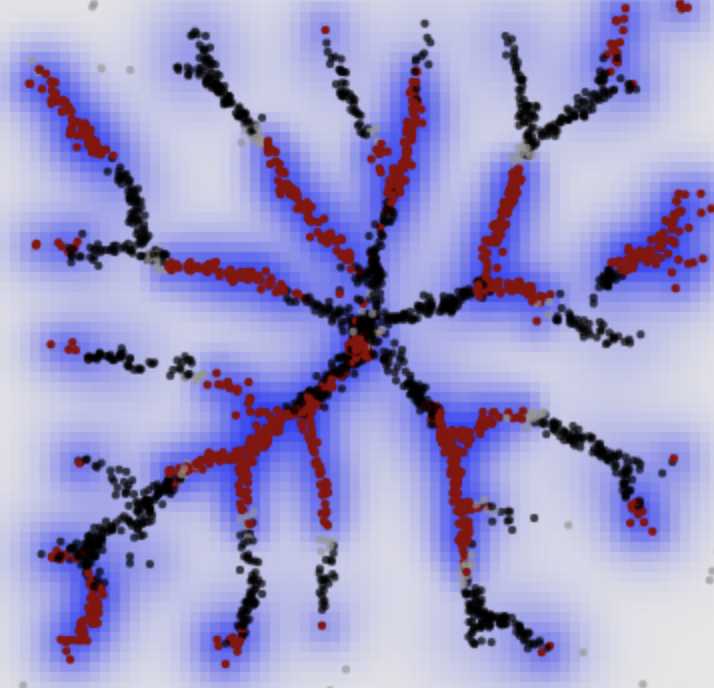
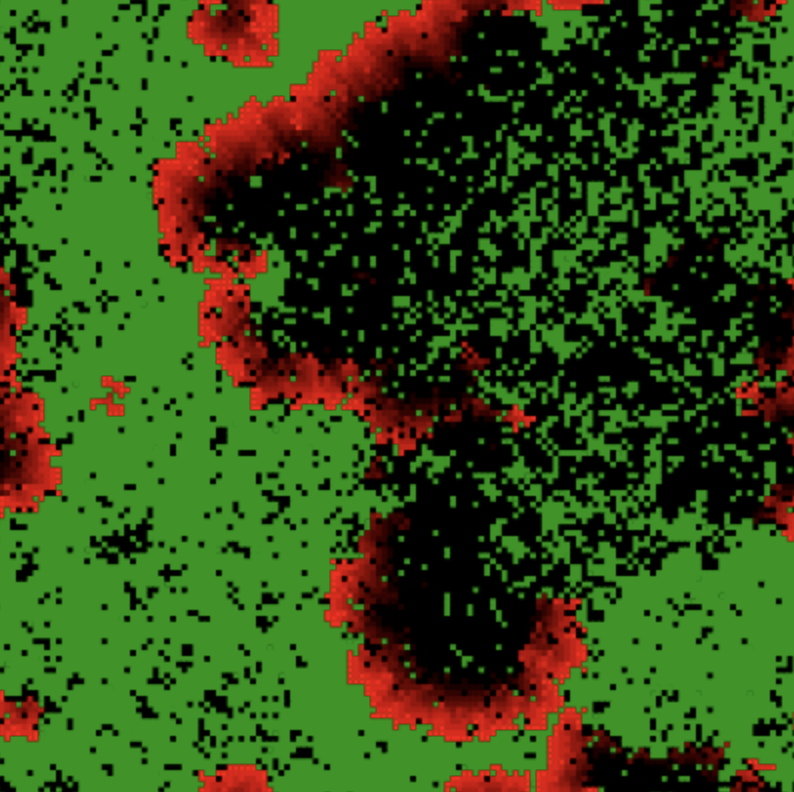
Chemotaxis in Dictyostelium discoideum
This explorable illustrates how simple, single-cell organisms can manage to aggregate into multi-cellular structures by emitting and responding to chemical signals. Individual cells respond by orienting towards a chemical signal and moving up its gradient, a process known as chemotaxis. The combination of synchronized signal emission and chemotaxis yields collective behavior with beautiful spatial branching patterns during the aggregation process.
“Nah dah dah nah nah... (Opus, 1984)”
Conway's Game of Life
“Hopfed Turingles”
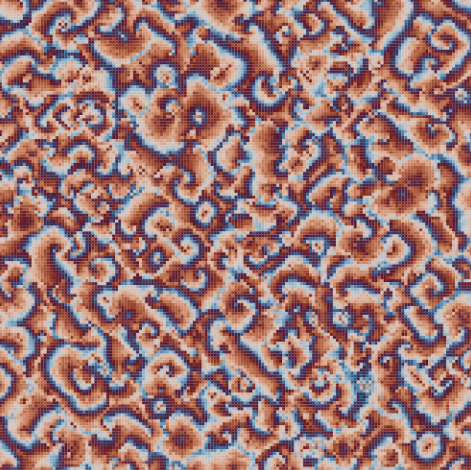
Pattern Formation in a simple reaction-diffusion system
With this explorable you can discover a variety of spatio-temporal patterns that can be generated with a very famous and simple autocatalytic reaction diffusion system known as the Gray-Scott model. In the model two substances $U$ and $V$ interact and diffuse in a two-dimensional container. Although only two types of simple reactions occur, the system generates a wealth of different stable and dynamic spatio-temporal patterns depending on system parameters.
“Critically Inflammatory”
A forrest fire model
This explorable illustrates, as a representative of a broad range of dynamic phenomena, a simple model for the spatial spread of forest fires and the dynamic patterns they generate. In the model two antagonistic processes interplay, the reproductive growth of vegetation that continuously covers the landscape with trees susceptible to fires and spontaneously seeded (lightning strikes) forest fires that spread across areas of dense vegetation.
“Dr. Fibryll & Mr. Glyde”
Pulse-coupled oscillators
This explorable illustrates pattern formation in excitable media. The example explored here in a system of pulse-coupled oscillators that are arranged on a two-dimensional lattice and interact with their neighbors by delivering excitatory pulses to them and receiving them in return. This model is sometimes used to study synchronization and can capture the dynamics of activation in layers of neurons as well as the spatial patterns of signaling molecules that play a role in microbial aggregation processes.
“Scott's World*”
Microbial growth patterns
This explorable illustrates a dynamic model for pattern formation in a growing community of microbes. Many microbial organisms exhibit collective behavior when a community of them expands say on a surface with nutrients. These patterns are often very beautiful and rich in structure.
“Surfing a Gene Pool”
Expansion of clones with idential fitness
This explorable is about pattern formation in a model system for the growth of a bacterial population in a petri dish in which the bacterial population is made up of a mix of two or three mutant strains that have identical fitness and reproduction properties. In the model system, an initially well mixed drop of bacteria with an equal amounts of every mutant strain is positioned in the center of the petri dish. After that, the bacteria start replicating, the population expands radially and a pattern will emerge.
“If you ask your XY”
The XY model of statistical mechanics
This explorable illustrates pattern formation and dynamics in the $XY$-model, an important model in statistical mechanics for studying phase-transitions and other phenomena. It’s a generalization of the famous Ising-Model. The $XY$-model is actually quite simple.
“Critical HexSIRSize”
The stochastic, spatial SIRS model
This explorable illustrates the behavior of a contagion process near its critical point. Contagion processes, for example transmissible infectious diseases, typically exhibit a critical point, a threshold below which the disease will die out, and above which the disease is sustained in a population. Interesting dynamical things happen when the system is near its critical point.
“Flock'n Roll”
Collective behavior and swarming
This explorable illustrates of an intuitive dynamic model for collective motion (swarming) in animal groups. The model can be used to describe collective behavior observed in schooling fish or flocking birds, for example. The details of the model are described in a 2002 paper by Iain Couzin and colleagues.
“Particularly Stuck”
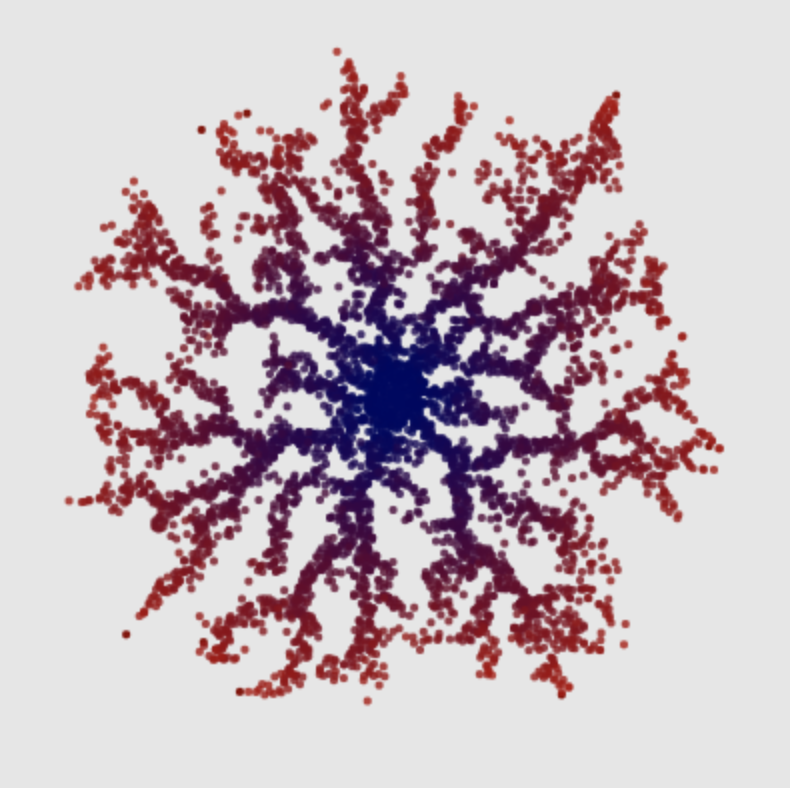
Diffusion Limited Aggregation
This explorable illustrates a process known as diffusion-limited aggregation (DLA). It’s a kinetic process driven by randomly diffusing particles that gives rise to fractal structures, reminiscent of things we see in natural systems. The process has been investigated in a number of scientific studies, e.g. the seminal paper by Witten & Sander.
“Cycledelic”
The spatial rock-paper-scissors game
This explorable of a pattern forming system is derived from a model that was designed to understand co-existance of cyclicly interacting species in a spatially extended model ecosystem. Despite its simplicity, it can generate a rich set of complex spatio-temporal patterns depending on the choice of parameters and initial conditions.
“Keith Haring's Mexican Hat”
Pattern Formation by Local Excitation and Long-Range Inhibition
This explorable illustrates one of the most basic mechanisms for spontaneous pattern formation: Local excitation and long range inhibition. This mechanism or similar ones are responsible for patterns observed in many natural systems, such as neural tissue, animal fur and spatial heterogeneity in social systems.